
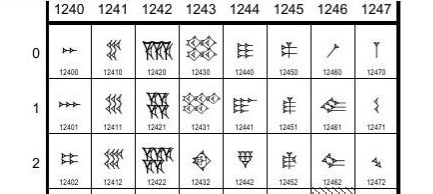
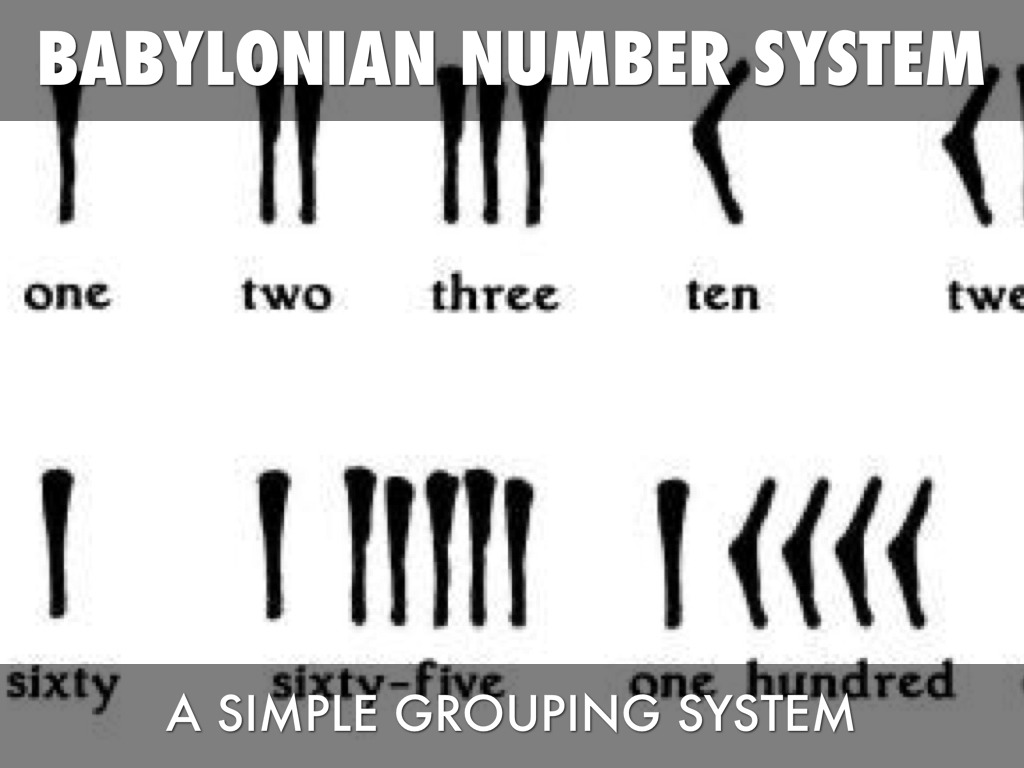
Sixty one is sixty and one, which therefore looks like one and one, and so on. The symbol for sixty seems to be exactly the same as that for one. However, something strange happened at sixty (see below). Eleven was ten and one, twelve was ten and one and one, twenty was ten and ten, just like the Egyptians. Once they got to ten, there were too many symbols, so they turned the stylus on its side to make a different symbol. However, they tended to arrange the symbols into neat piles. Like the Egyptians, the Babylonians used two ones to represent two, three ones for three, and so on, up to nine. I am using a yellow background to represent the clay!Įnter a number from 1 to 99999 to see how the Babylonians would have written it, or enter a number to count with. This explains why the symbol for one was not just a single line, like most systems. The Babylonians writing and number system was done using a stylus which they dug into a clay tablet. Eventually it was replaced by Arabic numbers. It is quite a complicated system, but it was used by other cultures, such as the Greeks, as it had advantages over their own systems. It started about 1900 BC to 1800 BC but it was developed from a number system belonging to a much older civilisation called the Sumerians. The digit is now clustered in the process.The Babylonian number system is old. Right then, you might want to try it out on the tool above to see other sequences. It has that right to left writing system feeling, but not really. We directly use 10 3 for 1,000 for example, but not in Greek numeral. We don't need to group it, it doesn't have myriad part.Īs you probably notice, the power (exponent) notation is the opposite version of what we use today. The multiplier sequence for Myriad: thousands ► hundreds ► tens ► ones.

Pay attention on how the grouping technique works for letter surrounding the M (10,000) above. (one hundred fifty two thousand and one hundred one)Īlso can be written as M ιε͵βραʹ (without the exponent in front of M, semi-formatted). (one hundred billion, ten mullion, one thousand and eleven)Īlso can be written as M ͵αM ͵α͵αιαʹ (without the exponent in front of M, semi-formatted).
Substitute each letter with its value from the table: There's β (2) in front of first M and α (1) in front of second M. Like 1x or 1a is written as x or a, sort of.Īnd for formatted (using pen and paper) writing, the exponent before M can be omitted. If there's one M and no letter written before it (on left side), meaning 10,000 powered by α (1).
Keep in mind, if there's M, then the letter before M will be used as the exponent. If the value is one hundred million, for instance 100,000,000 ► βMαʹ (beta myriad alpha) ► 10,000 2 × 1 = 100,000,000.īecause this isn't using pen and paper, let's try to read the un-formatted number. The alpha before the Myriad is the exponent or power, in this case 1 (α). This one just rises the numbers position a bit and put color on them so that you know it's the Myriad part. The format for Myriad is usually using that superscript writing format with the numbers (multipliers) are above the M letter. So, after 9,999 is Myriad (M) or alpha Myriad (αM). They had a letter representation for each number until 9,000. The Greek numeral system in that time was not exactly like in our period. It's not like M in Roman, which is 1,000. And to make it different than just letter (to form a word or not, but not number), it has that symbol that looks like an apostrophe but it's not, behind the number sequence.Īnd there's the M, as in Myriad, for the power of 10,000. It's kinda not like today's numeral system (modern Arabic - straight forward 0-9 decimal), and it's using Greek letter (numeral).


 0 kommentar(er)
0 kommentar(er)
